Map Projection Material: Definition, Type, Purpose, Examples

In general, map projection is a process of moving the parallels and meridians of the earth mathematically and systematically towards the representation of a grid shape in a flat plane.

A projection system on this one will not be able to perfectly carry out the transfer.

The selection in the projection system that will be used is made based on the original characteristics that will be preserved, the size and shape of the mapped area and its location on the earth's surface.

For more information regarding map projections, please read the reviews below.

Definition of Map Projection

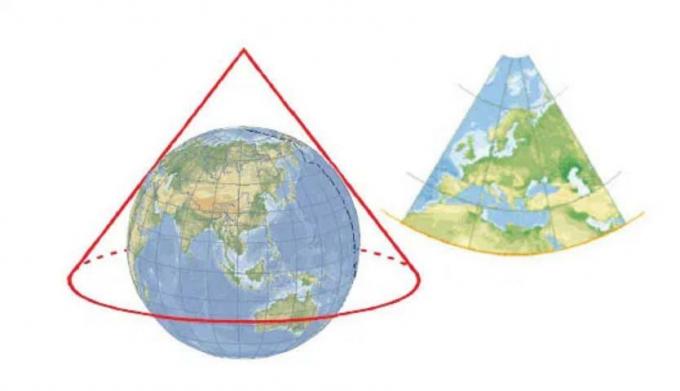
When viewed in general terms, map projection can be interpreted as a science that studies how to transfer topographic data from the Earth's surface towards the top of the map surface (flat plane), so that there are changes in shape, angle, area and changes distance.

However, there is also another definition which states that map projection is a function that relates the coordinates of existing points above the surface of a curve (generally in the form of an ellipsoid or a sphere) to the coordinates of the points above the plane flat.

Map Projection in Cartography

Map projection is one of the most important parts of cartography and the map-making process.

When viewed from the definition, cartography itself is a science and art related to mapping, be it for map-making or related to the map itself.

Meanwhile, the map is defined as an abstraction of objects and phenomena on the earth's surface that are selected, reduced, and drawn on a flat surface.

From this, it is known that the map has at least 4 keywords, including:

• Object abstraction.
• Minimized.
• Chosen.
• Drawn on a flat plane.

Map projection is also one of the key processes in cartography.

In a map projection in the field of cartography, it is necessary to depict a spherical surface of the earth on a flat plane.

From a technical point of view, map projection is done by moving the earth's nets into latitudes and longitudes on the map.

Map Projection Function

Map projection has a function to relate the coordinates of the points above the surface of a map curve (generally an ellipsoid or sphere) to the coordinates of the points above the plane flat.

That way, the process of transferring information related to the location of the earth's surface into the map will be greatly helped by the map projection.

And when using the right projection, map making can minimize or even eliminate errors or distortions that may occur during the map projection process.

Types of Map Projections

The following are several types of map projections which are divided into several categories, including:

a. Based on Intrinsic Element

If viewed from the intrinsic element, the projection system can be divided into:

• Preserved properties (area, distance, shape)
• Generation method and manufacturing method (geometric, mathematical)

b. Based on Extrinsic Element

Meanwhile, if based on extrinsic elements, the projection system can be divided into:

• Projection plane (planar plane, cone, cylinder)
• Projection plane position (transverse, normal).
• The tangent to the earth's axis (tangential, sectial).

c. By Generation or Method of Manufacture

When viewed from the generation or method of manufacture, it is divided into:

• Math (using calculations)
• Geometric (judging by the description).

d. Based on Preserved Original Traits

Then the projection system is further divided into:

• Maintain area (equivalent)
• Maintain distance (equidistant)
• Maintain shape (conform).

e. By Projection Field

Then the projection system is further divided into:

• Planar plane
• Cylinder
• Cone

f. Based on Projection Field Position

Then the projection system can be further divided into:

• Transverse (the axis of the projection plane is perpendicular to the axis of the earth)
• Normal (the axis of the plane of projection coincides with the axis of the earth)
• Oblique (axis of inclined plane of projection)

g. Based on Common Contact with Earth's Axis

When viewed from the intersection with the earth's axis, the projection system can be divided into:

• Tangential (projection plane tangent to the curvature of the earth)
• Secantial (projection plane that intersects the curvature of the earth)

In practice, each projection system can be divided according to all the criteria listed above.

For example:

The projection system that is very familiar and most widely used in Indonesia is the Universal Transverse Mercator (UTM) projection system.

Universal Transverse Mercator (UTM) projection system.

• Preserved trait: Shape or conformal.
• Projection plane: Cylinder.
• How to make: Mathematical.
• The tangent to the earth's axis: Secantial.
• Projection plane position: Transverse.

In general, each projection system has different characteristics or characteristics with different strengths and weaknesses.

Choosing a Map Projection System

Why is it necessary to choose a map projection and why does each region have a different projection system suitability?

This is caused by:

• Depending on the purpose of the map was created.
• The location and area of ​​the mapped area affect the selection of projections.
• Each projection system has different characteristics.

Then to choose the appropriate map projection system, knowledge of the basis for selecting the map projection itself is required.

The basis for selecting a map projection is that each transformation will have an effect on the representation of distance, direction, angle and area.

By having this knowledge, the selection of projections will be more appropriate. So that the mapping results will be more accurate in accordance with the mapping objectives.

The selection also requires adjusting the characteristics of the projection system for mapping purposes.

There are several factors that can influence the selection of map projections, including:

1. Mapping Purpose

Geographers as well as ecologists will focus on the relative size of the area.

Navigators, astronauts, meteorologists will also prioritize distances and angles.

The selection is based on several main aspects, for example on equivalent, conformity of form (conformal), azimuthal, fairness of appearance or others.

2. Distortion Values ​​and Settings

Some types of projections have specific patterns and settings for distortion. So that by knowing this, the selection of projections can be effective and optimal.

Conformity between the shape of the mapped area with the projection results is something that is desired.

This will be important when the map is created in series.

For serial maps, the selected projection should have the same distortion pattern in both large and small areas.

3. Overall Regional Shape

The shape of the area can be adjusted by using the size and format of the paper.

By using the correct projection, it is possible to display the map on a larger scale.

So that later a map with a lot of detail can be displayed more optimally.

Distortion in Map Projection

When viewed from the concept, map projection is done in 2 stages, namely:

• First, the earth is considered to be mapped on a globe (or so-called reference globe) which is scaled according to the scale of the map that will later be mapped.
• Second, move various points on the surface of the globe to a mathematically flat surface. So that a 3-dimensional surface can become a 2-dimensional surface.

There is no perfect projection system, that is, a projection system will not be able to perfectly carry out the transfer to a flat plane.

There are 4 types of distortion that can occur, including:

1. Angle Distortion

Angular distortion occurs when the angles of the lines on the map change when compared to the actual angles on the earth's surface.

The logic used to maintain the angular relationship is that each compass will point the same direction at each point on the earth (except the poles), that is, the division of the direction is always 90o.

If the direction is maintained, then the projection is conformal/orthomorphic.

It means maintaining existence.

The shape that is maintained only applies to a small area and the shape can change in other areas by a significant size.

2. Distance Distortion

Area distortion can occur when the distance between two points on the map changes when compared to the actual distance of the same point on the earth after scaling.

The problem of scale also needs to be considered in order to maintain the aspect of distance.

In order to obtain a distance that truly represents the actual distance between two points, the scale must be uniform along the boundary on the line joining the two points.

The scale must also be the same as the scale in the reference globe.

A map projection that maintains this aspect of distance is called a equidistant.

3. Directional Distortion

Directional distortion can occur when the direction on the map changes when compared to the actual direction on the earth's surface.

A map projection that maintains the directional aspect is called a azimuthal projection.

4. Distortion Area

Area distortion occurs when the relative area of ​​the map changes when compared to the actual area on the earth's surface.

Projections that retain a representation of the area (relatively area) are called equal-area or equivalent projections.

There are no projections which are conformal and equivalent projections at the same time.

This also indicates that all conformal projections will display the same area of ​​the earth with different sizes.

And all equivalent projections will later change most of the angles.

Map Projection Suitability based on Location

There is also a world map projection system that is often used, including:

• Mercator
• World Mercator
• Web Mercator

Meanwhile, on maps containing various countries and large areas, projections based on latitude can be used.

The map projection used to map the polar regions and high latitudes is a map projection that uses a flat plane as the projection area.

Or in other words it is often referred to as an azimutal projection.

For example the Lambert Equal Area projection, Azimutal Equidistant.

The correct map projection at temperate latitudes is a map projection in a conical projection plane. For example in polyconic projection, Lambert equal area.

Meanwhile, the appropriate map projection for mapping the equatorial region or low latitude area is a projection that is using cylindrical projection planes such as Mercator projection, Transverse Mercator and Universal Transverse Mercator (UTM).

A Map Projection System Suitable for Indonesia

Indonesia is a country located in the equatorial region or low latitude.

Therefore, the most appropriate projection is a projection with a tubular projection plane or a cylindrical projection.

Furthermore, the shape itself extends from west to east, so it is more appropriate if the projection axis is perpendicular to the earth's axis or transverse projection.

The projection that becomes the cylindrical projection and the transverse projection is the Transverse Mercator projection.

In order to further minimize distortion, because Indonesia is a large area, a sectial projection or a projection whose plane of projection intersects the surface of the earth itself.

One projection system that uses a cylindrical, transverse and sectial projection plane is the Universal Transverse Mercator (UTM).

So that it can be concluded that if you want to map all parts of the Indonesian state, then use a cylindrical projection system like the Mercator.

If you want to map narrower areas such as provinces and districts, you can use the Universal Transverse Mercator projection system/Transverse Mercator projection system.

Example of a Map Projection and its Image

The following are some examples of map projections and their images that you need to know, including:

1. Robinson's Projection

This one projection came out in 1961.

It differs only from the conformal equal area projection but is a combination of the two.

There is also a distinctive characteristic of this one projection, which is to distort the shape, area, scale and distance in an effort to balance the error nature of the projection.

Standard parallel lines will also be considered when the projection will show the Earth's surface on a small scale.

The advantage of using this projection system is that of the earth's surface can be depicted with a 20 percent degree of truth in its original scale.

2. Polyconic Projection

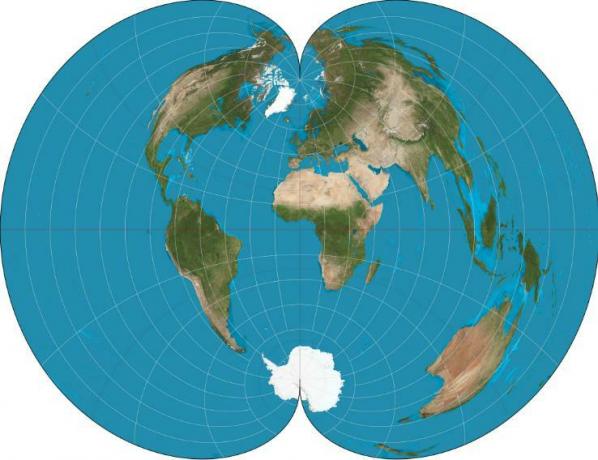
The larger the scale of the mapping, the more things that must be considered.

Such as:

• The similarity of form is an important factor.
• Overall distortion should be minimal.
• As well as various adjacent maps must be able to be juxtaposed precisely and accordingly.

This one polyconic projection was chosen to overcome this one thing

But this one projection will only produce a variety of adjacent maps that can be juxtaposed in certain directions and not in other directions.

3. Mercator Projection

The mercator projection uses a cylindrical shape to become the projection plane.

In this projection, all the rhumb lines appear as straight lines.

Meanwhile, the lines from the great circle do not look straight, except in the equator and meridians.

In a normal mercator projection, the standard line is the equator. Which along the line has a scale factor = 1.0.

The value of the scale factor changes only very slightly around the equator. So this one projection will be very fitting for the area at the equator.

4. Transverse Mercator Projection

The transverse mercator projection is a mercator projection in which the projection plane is rotated 90 degrees.

So the standard lines are meridians, not parallels.

This one projection will be very suitable for use in a small area in the standard meridian area.

In this one projection, it is also widely used for topographic mapping, and has become the basis of the Universal Transverse Mercator (UTM) coordinate system.

5. Albers Equal Area Projection

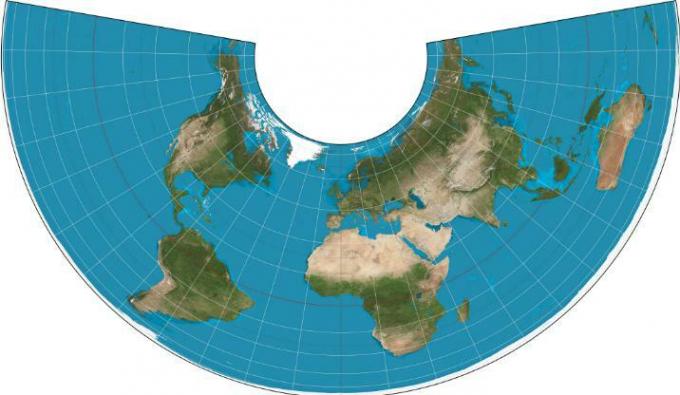
This one system uses two parallel standards.

2 Adjacent small circles can be selected to be the standard parallel.

The closer the two lines are, the better the representation in the area around the line directly.

This system has a very low distortion value.

Its appearance resembles the earth's graticule, namely straight meridians converging and parallel curved concentrically and intersecting with meridians at right angles.

Outside of standard parallels, the value of the scale factor decreases, while among standard parallels, the value of the scale factor increases.

This one projection is very appropriate for use in areas in the middle latitudes which have a larger east-west boundary than the north-south boundary.

6. Lamberts Equal Area Projection

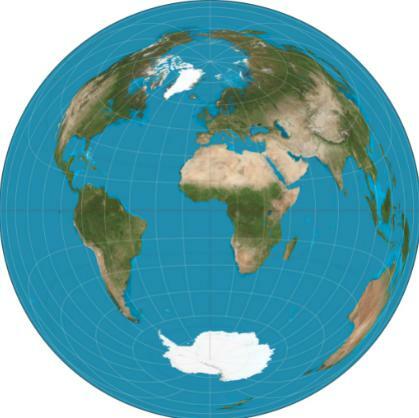
This one projection is very commonly used, including the azimuthal equivalent.

Symmetrical distortion that surrounds a central point, and can be located anywhere.

Therefore, projections are very useful in areas that extend east-west and have north-south dimensions.

7. Space Oblique Mercator Projection

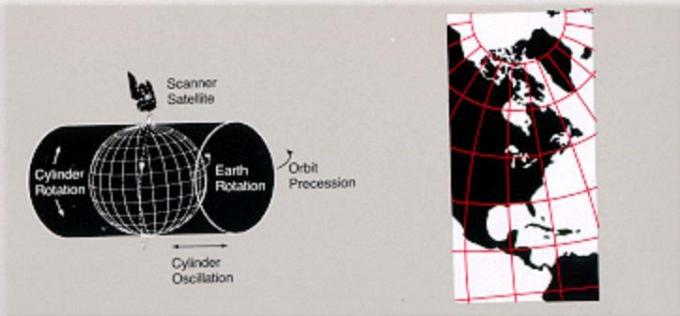
This one projection will appear as a response to the presence of satellite imagery from remote sensing.

In this one projection, it also becomes a Mercator projection where the standard line is created obliquely following the trajectory of the satellite.

It will also make geometric correction errors in the image to a minimum.

This projection system has conformal properties.

8. Universal Transverse Mercator (UTM) Projection

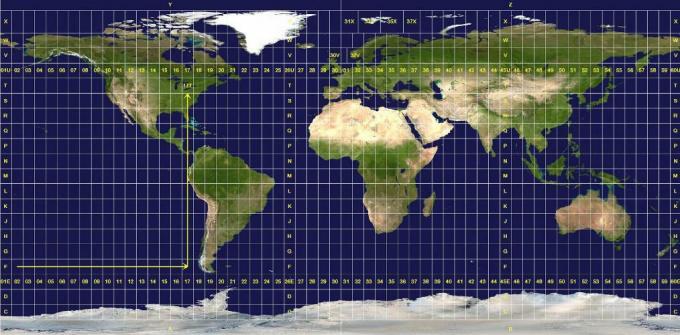
The Universal Transverse Mercator (UTM) projection system is a projection system in the plane cylindrical projection, the position of the projection plane is transverse, and will touch the earth's surface (sectional).

This one projection will be very fitting if used in the equatorial region, such as in Indonesia.

The RBI map issued by BIG has also used this projection system.