The formula for the perimeter of a trapezoid with examples of the problem
Formula.co.id – After previously we discussed about area formula for cone this time we will discuss the material about the formula for the perimeter of a trapezoid, we will describe it in detail and complete from the understanding of the trapezoid, the properties of the trapezoid, the kinds, formulas, and examples of problems from the circumference trapezoid.

Table of contents :
Definition of Trapezoid
What is a Trapezoid? you already know, Trapezoid is a two-dimensional flat shape which is also formed by 4 edges which are parallel but not the same length.
Trapezoidal Properties
Trapezoid also has its own properties, namely:
- A trapezoid has 4 sides and 4 vertices.
- Trapezoid also has a pair of sides that are very parallel but not too long.
- A trapezoid has an angle between which the parallel sides are 180o.
Types of Trapezoid
Based on the length of the legs, trapezoids can also be divided into several types, namely:
1. Any Trapezoid:

The above trapezoid ABCD, AB // DC, has unequal lengths ( AD BC ) and neither legs are perpendicular to the parallel sides. Such a trapezoid can be called any trapezoid.
2. Elbow Trapezoid - Elbow:
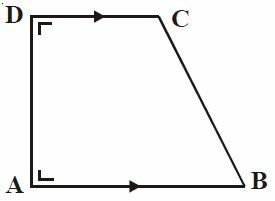
The above trapezoid ABCD shows that one of its legs is perpendicular to its parallel sides, namely AD ^ AB and AD ^ DC. Trapezoid like this is called a right-angled trapezoid.
3. Isosceles Trapezoid:

The trapezoid ABCD above has legs of the same length, namely AD and BC. Such a trapezoid is called isosceles trapezoid.
Trapezoidal Formula
The formula for the perimeter of a trapezoid is as follows:
K = sum of all sides (eg AB+BC+CD+DA)
The formula for the area of a trapezoid is as follows:
L = x number of parallel sides x height
Examples of Trapezoidal Problems
Example Question 1
1. If a trapezium has sides AB = 2 cm, BC = 7 cm, CD = 9 cm, DA = 8 cm, find and calculate the perimeter of the trapezium!
Solution:
Is known:
- Side AB = 2 cm,
- Side BC = 7 cm
- CD side = 9 cm
- Side DA = 8 cm
Asked: K = …?
Answer:
- K = AB + BC + CD + DA
- L = 2cm + 7cm + 9cm + 8cm
- L = 26 cm
So, The perimeter of the trapezium is = 26 cm
Example Question 2
2. If a trapezium has side lengths AB = 32 cm, BC = 27 cm, CD = 19 cm, DA = 18 cm, find and calculate the perimeter of the trapezium!
Solution:
Is known:
- Side AB = 32 cm,
- Side BC = 27 cm
- CD side = 19 cm
- Side DA = 18 cm
Asked: K = …?
Answer:
- K = AB + BC + CD + DA
- L = 32cm + 27cm + 19cm + 18cm
- L = 96 cm
So, The perimeter of the trapezium is = 96 cm
Example Question 3
3. If a trapezium has side lengths AB = 27 cm, BC = 56 cm, CD = 69 cm, DA = 33 cm. Find and calculate the perimeter of the trapezium!
Solution:
Is known:
- Side AB = 27 cm,
- Side BC = 56 cm
- CD side = 69 cm
- Side DA = 33 cm
Asked: K = …?
Answer:
- K = AB + BC + CD + DA
- L=27cm+56cm+69cm+33cm
- L = 185 cm
So, The perimeter of the trapezium is = 185 cm
Example Question 4
4. If a trapezium has side lengths AB = 66 cm, BC = 47 cm, CD = 44 cm, DA = 80 cm, find and calculate the perimeter of the trapezium!
Solution:
Is known:
- Side AB = 66 cm,
- Side BC = 47 cm
- CD side = 44 cm
- Side DA = 80 cm
Asked: K = …?
Answer:
- K = AB + BC + CD + DA
- L = 66cm + 47cm + 44cm + 80cm
- L = 237 cm
So, The perimeter of the trapezium is = 237 cm
Example Question 5
5. If a trapezium has side lengths AB = 5 cm, BC = 3 cm, CD = 7 cm, DA = 10 cm, find and calculate the perimeter of the trapezium!
Solution:
Is known:
- Side AB = 5 cm,
- Side BC = 3 cm
- CD side = 7 cm
- Side DA = 10 cm
Asked: K = …?
Answer:
- K = AB + BC + CD + DA
- L = 5cm + 3cm + 7cm + 10cm
- L = 25 cm
So, The perimeter of the trapezoid is = 25 cm
This is a complete discussion on how to calculate the formula for the perimeter of a trapezoid along with examples of questions and their discussion, hopefully it will be useful…
Related Formulas:
- Circumference of a Semicircle
- The formula for the circumference of a rhombus
